
by Jean Barbet | Jun 8, 2024 | Logic, Set Theory
Based on the notions of object and class derived from natural logic, we have redefined the concept of set in an intuitive way, thus establishing a natural set theory without resorting to formal logic. This approach is based on six axioms that form the basis of this...

by Jean Barbet | May 24, 2024 | Logic, Set Theory
The revolution in mathematics is that of set theory, which responds both to the problem of a universal and rigorous conceptual language and to that of a single foundation for all mathematical disciplines. Although set theory was Cantor’s original work, the...

by Jean Barbet | Jul 9, 2023 | Logic, Number Theory, Set Theory
Natural arithmetic is the science of natural numbers: it is based on addition, multiplication, natural order and divisibility. Now, all these operations and relations are defined on the basis of the single successor function, whose properties are brought together in...
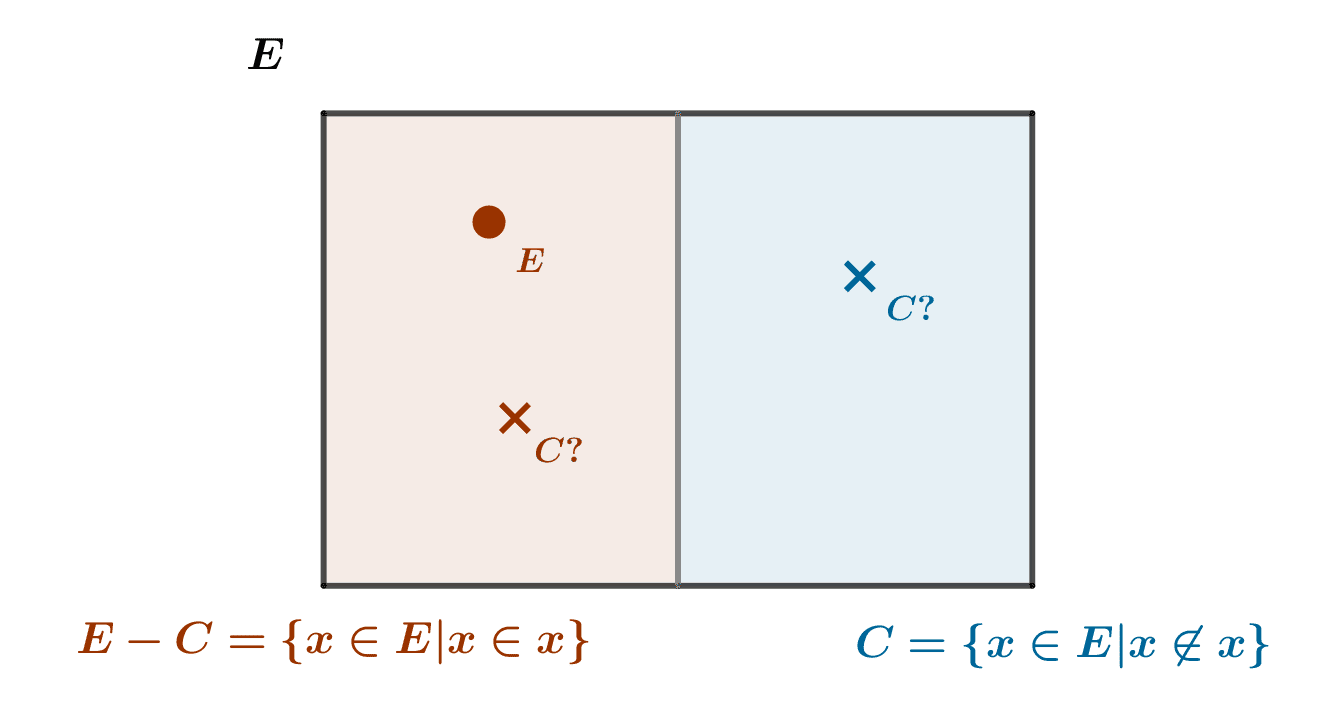
by Jean Barbet | May 29, 2021 | Logic, Set Theory
Russell’s paradox or antinomy is a very simple paradox in naive set theory, which arises when one tries to define a “set of all sets”. Its resolution relies on the introduction of the notion of class and the distinction of sets among classes. Thanks...
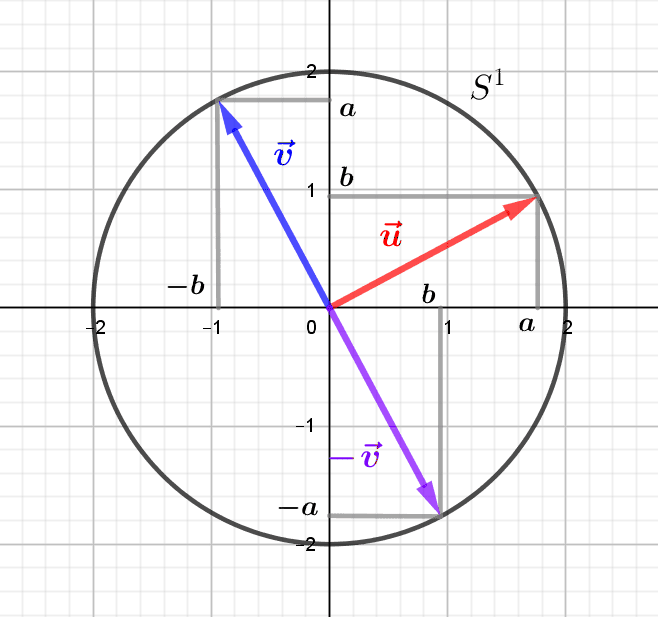
by Jean Barbet | May 23, 2021 | Algebra, Geometry
The linear transformations of the Euclidean plane are the invertible linear applications, i.e. of non-zero determinant. They allow us to move from one basis of the plane to another, and the orthogonal transformations, i.e. the vectorial isometries, exchange the...






